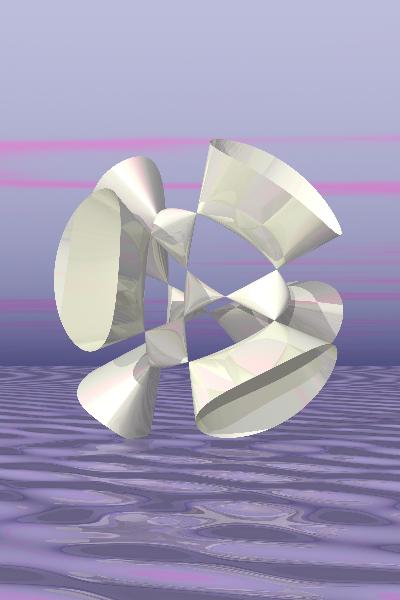

This is a picture of Kummer's surface, an algebraic surface defined by a polynomial equation of degree four. It has sixteen double points, the maximum possible for a surface of degree four in three-dimensional space. All sixteen points appear in this picture, as singularities at the vertices of the five tetrahedra.
The picture was produced by ray-tracing, a technique based on following the paths of light rays through the scene.
Another picture of the surface is available.
A very detailed treatment of Kummer's surface appears in R.W.H.T. Hudson's Kummer's Quartic Surface, Cambridge University Press 1905.